A-Math - Integration -
Differentiating and Integrating to Find an Expression
 |
| Topic: Integration |
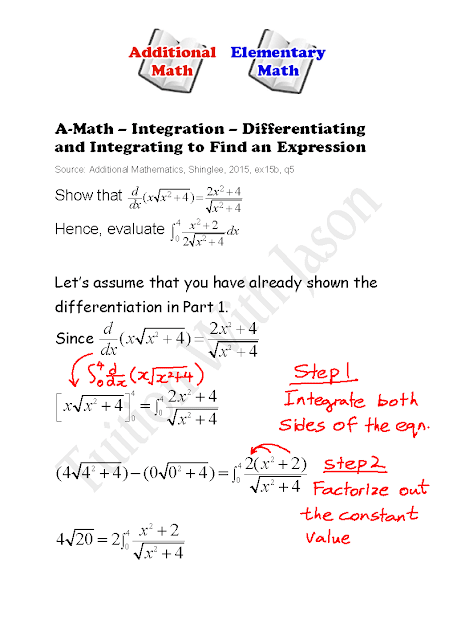
I did not work out the first part of the problem, you can try to prove/show
it on your own. Here is a clue for those who are unsure on how to do,use
change \((x\sqrt {{x^2} + 4} )\) to \(x{({x^2} + 4)^{\frac{1}{2}}}\)
and use product rule to differentiate.
In the second part of the problem, the first step is to integrate both sides
of the equation, do note that when you integrate \(\frac{d}{{dx}}(x\sqrt {{x^2} + 4} )\)
you will get \((x\sqrt {{x^2} + 4} )\) as differentiation and integration
cancels out each other since they are the opposite of each other. You
have to use the answer in part one to integrate part two since there is
no formula to integrate an algebric fraction like the one above, unlike
differentiation where we can use the quotient rule.




Blogger Comment
Facebook Comment