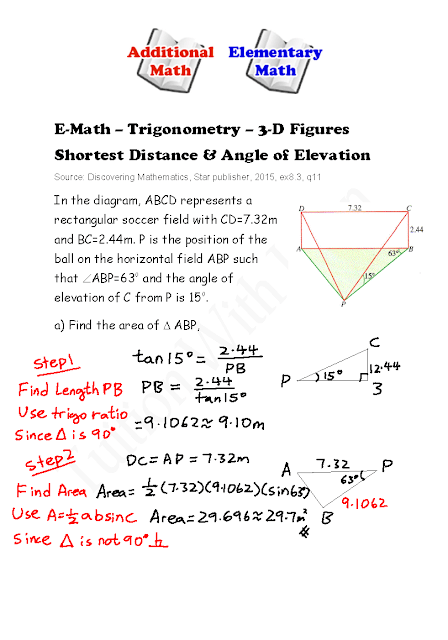
E-Math - Trigonometry - 3-D Figures Shortest Distance and Angle of Elevation

E-Math - Trigonometry - 3-D Figures 

Shortest Distance and Angle of Elevation

Topic:Trigonometry

If you have difficulty visualizing the trigonometry 3-D figure, you
could draw out the portion of the figure that you are solving, it only
takes a matter of seconds to draw the triangles. A few seconds lost
in a test or exam is much better then losing a few marks.

Part a) you may assume that any vertical object is \( \bot \) or 90
degrees to the horizontal , in this case the goal post is \( \bot \)
to the field. As such, you may use trigonometric ratio i.e.tangent, sine
and cosine.

Part b) I repeat that the shortest distance from a line to a point, is a
\( \bot \) line from the line to the point. Draw this line to form a
Right angle Triangle, hen you may use trigo ratio once more to solve.

Note: Sine Rule \(\frac{a}{{\sin A}} = \frac{b}{{\sin B}}\) and
Cosine Rule \({c^2} = {a^2} + {b^2} - 2ab\;{\mathop{\rm Cos}\nolimits} C\)
are used for NON right angle triangles. In deciding which one to use, just
remember that for Sine Rule you need to know the value of one of its angles
and the value of the corresponding side i.e. the side opposite the angle.

Share on Google Plus

About Unknown

I created this Blog after my students told me that there is very little information about how to solve GCE O Level Math and Additional Math (A-Math) questions on the internet. Even if they manage to find, the solution were for very basic questions. I am a Full-time Math Tutor in Singapore. I tutor mainly in Northern Singapore (Woodlands, Chua Chu Kang, Bukit Panjang, Yishun and Sembawang). I also tutor in Johor Bahru.